


Next: Treatment of proton and
Up: Approximations to ATDHFB
Previous: Perturbative cranking approximation
Gaussian overlap approximation
To compare cranking expressions with those obtained within the GOA, it is
convenient to introduce the 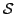 matrices [23]:
matrices [23]:
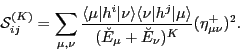 |
(61) |
It is immediately seen that for the mass tensor of Eq. (60)
one has
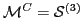 . In the case of GOA, also
assuming weak state dependence of pairing and neglecting the
derivatives of
. In the case of GOA, also
assuming weak state dependence of pairing and neglecting the
derivatives of  and
and
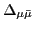 , one obtains
[24,23]
, one obtains
[24,23]
![\begin{displaymath}
{\cal M}^{\rm GOA}=
{\cal S}^{(2)}\left[{\cal S}^{(1)}\right]^{-1}{\cal S}^{(2)}.
\end{displaymath}](img172.png) |
(62) |
Evaluating the matrix elements of 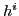 entering Eq. (61)
perturbatively, one can express
entering Eq. (61)
perturbatively, one can express 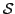 explicitly through the
matrix elements of the constraining field operators
explicitly through the
matrix elements of the constraining field operators 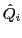 :
:
![\begin{displaymath}
{\cal S}^{(K)}=\frac{1}{4}\left[{ M}^{(1)}\right]^{-1}{ M}^{(K)}
\left[{ M}^{(1)}\right]^{-1},
\end{displaymath}](img175.png) |
(63) |
where the energy-weighted moments 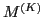 are given by
are given by
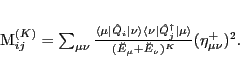 |
(64) |
Jacek Dobaczewski
2010-07-28
![]() matrices [23]:
matrices [23]: