The survey of ISB corrections in
| Parent |
|
|
|
|
|
|
|
|
||||||
| nucleus | (s) | (%) | (%) | (%) | (%) | (s) | (%) | (%) | (s) | |||||
| 3041.7(43) | 0.559 | 0.559 | 0.823 | 0.65(14) | 3062.1(62) | 0.37(15) | 3.7 | 0.462(65) | 3067.8(49) | |||||
| 3042.3(11) | 0.303 | 0.303 | 0.303 | 0.303(30) | 3072.3(21) | 0.36(06) | 0.8 | 0.480(48) | 3066.9(24) | |||||
| 3052.0(70) | 0.243 | 0.243 | 0.417 | 0.301(87) | 3080.5(75) | 0.62(23) | 1.9 | 0.342(49) | 3079.2(72) | |||||
| 3052.7(82) | 0.865 | 0.997 | 1.475 | 1.11(29) | 3056(12) | 0.63(27) | 3.1 | 1.08(42) | 3057(15) | |||||
| 3036.9(09) | 0.308 | 0.308 | 0.494 | 0.370(95) | 3070.5(31) | 0.37(04) | 0.0 | 0.307(62) | 3072.5(23) | |||||
| 3049.4(11) | 0.809 | 0.679 | 1.504 | 1.00(38) | 3060(12) | 0.65(05) | 48.4 | 0.83(50) | 3065(15) | |||||
| 3047.6(12) | -- | -- | -- | 0.77(27) | 3069.2(85) | 0.72(06) | 0.5 | 0.70(32) | 3071(10) | |||||
| 3049.5(08) | 0.486 | 0.486 | 0.759 | 0.58(14) | 3074.6(47) | 0.71(06) | 4.5 | 0.375(96) | 3080.9(35) | |||||
| 3048.4(07) | 0.460 | 0.460 | 0.740 | 0.55(14) | 3074.1(47) | 0.67(07) | 3.1 | 0.39(13) | 3079.2(45) | |||||
| 3050.8(10) | 0.622 | 0.622 | 0.671 | 0.638(68) | 3074.0(32) | 0.75(08) | 2.0 | 0.51(20) | 3078.0(66) | |||||
| 3074.1(11) | 0.925 | 0.840 | 0.881 | 0.882(95) | 3090.0(42) | 1.51(09) | 44.0 | 0.49(11) | 3102.3(45) | |||||
| 3084.9(77) | 2.054 | 1.995 | 1.273 | 1.77(40) | 3073(15) | 1.86(27) | 0.1 | 0.90(22) | 3101(11) | |||||
|
|
3073.6(12) | 112.2 |
|
3075.0(12) | ||||||||||
|
|
0.97397(27) |
|
10.2 |
|
0.97374(27) | |||||||||
| 0.99935(67) | 0.99890(67) |
| Parent |
|
|
|
|
|
||
| nucleus | (%) | (%) | (%) | (%) | (%) | ||
| 2.031 | 1.064 | 1.142 | 1.41(46) | 0.72(30) | |||
| 0.399 | 0.399 | 0.597 | 0.47(10) | 0.529(77) | |||
| 1.731 | 1.260 | 1.272 | 1.42(26) | 0.98(21) | |||
| 1.819 | 0.956 | 0.987 | 1.25(42) | 0.42(24) | |||
| 0.255 | 0.255 | 0.535 | 0.35(14) | 0.216(86) | |||
| 1.506 | 0.974 | 1.009 | 1.16(27) | 0.60(20) | |||
| 0.956 | 0.925 | 1.694 | 1.19(38) | 0.64(12) | |||
| 1.654 | 1.479 | 1.429 | 1.52(18) | 1.10(52) |
The results of our calculations are collected in Tables 2
and 3, and in Fig. 6. In addition,
Fig. 7 shows the differences,
![]() , between our results and those of
Ref. [3]. In spite of clear differences between SV and HT, which can be seen for specific transitions including those for
, between our results and those of
Ref. [3]. In spite of clear differences between SV and HT, which can be seen for specific transitions including those for
![]() , 34, and 62, both calculations reveal a similar
increase of
, 34, and 62, both calculations reveal a similar
increase of
![]() versus
versus ![]() , at variance with the RPA calculations of
Ref. [13], which also yield systematically smaller values.
, at variance with the RPA calculations of
Ref. [13], which also yield systematically smaller values.
![\includegraphics[angle=0,width=0.8\columnwidth]{deltaC.fig06.eps}](img218.png) |
![\includegraphics[angle=0,width=0.7\columnwidth,clip]{deltaC.fig07.eps}](img220.png) |
The ISB corrections used for further calculations
of
![]() are collected in Table 2.
Let us recall that our preference is to use the
averaged corrections and that the
are collected in Table 2.
Let us recall that our preference is to use the
averaged corrections and that the ![]() K
K
![]()
![]() Ar transition has been disregarded.
All other ingredients needed to compute the
Ar transition has been disregarded.
All other ingredients needed to compute the ![]() -values,
including radiative
corrections
-values,
including radiative
corrections
![]() and
and
![]() , are taken
from Ref. [3], and the empirical
, are taken
from Ref. [3], and the empirical ![]() -values are taken from
Ref. [5]. For the sake of completeness, these empirical
-values are taken from
Ref. [5]. For the sake of completeness, these empirical
![]() -values are also listed in Table 2.
-values are also listed in Table 2.
![\includegraphics[width=0.9\columnwidth]{deltaC.fig08.eps}](img223.png) .
. |
In the
error budget of the
resulting ![]() -values listed in Table 2,
apart from errors in the
-values listed in Table 2,
apart from errors in the ![]() values
and radiative corrections, we also included the uncertainties estimated for
the calculated values of
values
and radiative corrections, we also included the uncertainties estimated for
the calculated values of
![]() , see Sec. 3.3.
To conform with HT, the average value
, see Sec. 3.3.
To conform with HT, the average value
![]() s was calculated by using the
Gaussian-distribution-weighted formula.
However, unlike HT, we do not apply any further corrections to
s was calculated by using the
Gaussian-distribution-weighted formula.
However, unlike HT, we do not apply any further corrections to
![]() .
This leads to
.
This leads to
![]() , which agrees very well
with both the HT result [3],
, which agrees very well
with both the HT result [3],
![]() ,
and the central value obtained from the neutron decay
,
and the central value obtained from the neutron decay
![]() [10].
A survey of the
[10].
A survey of the
![]() values deduced by using different methods is
given in Fig. 8. By combining the value of
values deduced by using different methods is
given in Fig. 8. By combining the value of
![]() calculated here with those of
calculated here with those of
![]() and
and
![]() of the 2010 Particle Data
Group [10], one obtains
of the 2010 Particle Data
Group [10], one obtains
It is worth noting that by using
![]() values corresponding to the fixed current-shape
orientations (
values corresponding to the fixed current-shape
orientations (
![]() ,
,
![]() , or
, or
![]() ) instead of their
average, one still obtains compatible results for
) instead of their
average, one still obtains compatible results for
![]() and unitarity condition (20), see Figs. 8
and 9. Moreover, the value of
and unitarity condition (20), see Figs. 8
and 9. Moreover, the value of
![]() obtained
by using SHZ2 is only
obtained
by using SHZ2 is only ![]() 0.024% smaller than
the SV result, see Table 2. This is an intriguing result, which indicates that an increase
of the bulk symmetry energy - that tends to restore the isospin
symmetry - is partly compensated by other effects. The most
likely origin of this compensation mechanism is due to the time-odd
spin-isospin mean fields, which are poorly constrained by the standard
fitting protocols of Skyrme EDFs [41,42,43].
For instance, if one compares the Landau-Migdal parameters characterizing the
spin-isospin time-odd channels [41,42,43] of SV (
0.024% smaller than
the SV result, see Table 2. This is an intriguing result, which indicates that an increase
of the bulk symmetry energy - that tends to restore the isospin
symmetry - is partly compensated by other effects. The most
likely origin of this compensation mechanism is due to the time-odd
spin-isospin mean fields, which are poorly constrained by the standard
fitting protocols of Skyrme EDFs [41,42,43].
For instance, if one compares the Landau-Migdal parameters characterizing the
spin-isospin time-odd channels [41,42,43] of SV (
![]() ,
,
![]() ,
,
![]() ,
,
![]() ) and SHZ2
(
) and SHZ2
(
![]() ,
,
![]() ,
,
![]() ,
,
![]() ) one notices that these two functionals differ by a factor of two in the scalar-isoscalar Landau-Migdal parameter
) one notices that these two functionals differ by a factor of two in the scalar-isoscalar Landau-Migdal parameter
![]() .
.
![\includegraphics[width=0.8\columnwidth]{deltaC.fig10.eps}](img245.png) |
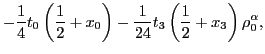 |
(21) | ||
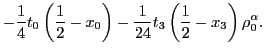 |
(22) |