


Next: Derivation of Eq. (98)
Up: Solution of self-consistent equations
Previous: Acknowledgements
Calculation of coefficients 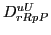 (72)
(72)
Coefficients 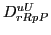 (72) can easily be calculated by using
the fact
that they exactly correspond to the multiplication of tensors
(72) can easily be calculated by using
the fact
that they exactly correspond to the multiplication of tensors 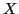 built
from the position vector
built
from the position vector 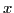 .
.
Tensors 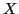 are simply related to spherical harmonics (see Eq. 5.2(40) in Ref. [6]):
are simply related to spherical harmonics (see Eq. 5.2(40) in Ref. [6]):
Therefore, multiplication law of spherical harmonics (Eq. 5.6(8) in Ref. [6]) gives
and thus the required coefficients read
and are independent of 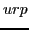 .
.



Next: Derivation of Eq. (98)
Up: Solution of self-consistent equations
Previous: Acknowledgements
Jacek Dobaczewski
2010-01-30
![]() (72) can easily be calculated by using
the fact
that they exactly correspond to the multiplication of tensors
(72) can easily be calculated by using
the fact
that they exactly correspond to the multiplication of tensors ![]() built
from the position vector
built
from the position vector ![]() .
.