


Next: Bibliography
Up: Particle-Number Projection and the
Previous: Deformation energy within the
Conclusions
This study contains the systematic analysis of the particle-number-projected
DFT approach. This approach, usually in the Skyrme-HFB or
Gogny-HFB framework, is commonly used in systematic calculations of
nuclear ground-state properties, low-energy excitations, and high-spin
states. For heavy, complex nuclei, the nuclear DFT is the only viable
microscopic tool based on the effective interaction (or functional). To
advance the nuclear DFT further, to improve its theoretical foundations,
and to make it a more reliable tool, it is important to fully understand
the advantages and drawbacks of the method when applied to self-bound
nuclear systems.
The main conclusions of this work can be summarized as follows:
- The transition density matrices connecting states having different
orientation in the gauge plane
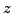 have poles on the imaginary axis
have poles on the imaginary axis
![${\mbox{\rm\scriptsize {Im}}[z]}$](img271.png) .
In the HFB formalism that is based on one Hamiltonian acting in all channels
(Hamiltonian-based HFB), these poles are irrelevant as their impact
is nullified by the cancellation between the Hamiltonian matrix elements
originating from particle-hole and pairing channels. Such a cancellation
is not present in the HFB applications in which some of
the Hamiltonian matrix elements are neglected (or approximated),
in the HFB method based on density-dependent interactions (usually
acting in different channels), and
in the DFT approach in which the Hamiltonian does not appear at all.
In all those cases, the projection operator is not defined uniquely
and the result depends on the analytic structure of the transition energy
density; hence, the projected DFT energy depends on
the integration contour. The resulting PNP energy can be expressed in terms of
individual residues corresponding to the poles
.
In the HFB formalism that is based on one Hamiltonian acting in all channels
(Hamiltonian-based HFB), these poles are irrelevant as their impact
is nullified by the cancellation between the Hamiltonian matrix elements
originating from particle-hole and pairing channels. Such a cancellation
is not present in the HFB applications in which some of
the Hamiltonian matrix elements are neglected (or approximated),
in the HFB method based on density-dependent interactions (usually
acting in different channels), and
in the DFT approach in which the Hamiltonian does not appear at all.
In all those cases, the projection operator is not defined uniquely
and the result depends on the analytic structure of the transition energy
density; hence, the projected DFT energy depends on
the integration contour. The resulting PNP energy can be expressed in terms of
individual residues corresponding to the poles 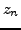 associated
with single-particle (canonical) proton and neutron orbits. In contrast,
in the Hamiltonian-based HFB, the result depends only
on the single pole at the origin (
associated
with single-particle (canonical) proton and neutron orbits. In contrast,
in the Hamiltonian-based HFB, the result depends only
on the single pole at the origin (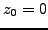 ).
).
- Within the Hamiltonian-based HFB, there exist sum rules
that relate the unprojected
matrix elements with the matrix elements in the projected states. Similar
sum rules can be derived within the DFT; they relate the unprojected DFT
transition energy density with the projected DFT energies.
The DFT sum rules offer the
interpretation of the projected DFT energies as Fourier components
(associated with irreducible representations of gauge group U(1))
of the DFT transition energy density. This can be naturally
extended to other (higher) broken symmetry groups, such as SU(2) (associated
with the broken angular momentum symmetry).
- The discussion of the particle number restoration
can be extended to other symmetry restoration problems.
In particular,
DFT transition densities associated with angular-momentum-projected states
are expected to have complicated pole
structure in the three dimensional space of Euler angles (see the
example shown in Ref. [45]).
- For the terms in the density functional that have polynomial density
dependence, the appearance of poles inside the contour gives rise to
sudden jumps of the projected energy whenever the contour's pole content
changes. Otherwise, the results are stable. This is not true for the
terms having fractional-power density dependence (e.g.,
density-dependent pieces of many Skyrme and Gogny interactions or the
Coulomb exchange term taken in the Slater approximation). Here, the
dependence on the contour radius shows a strong subthreshold behavior
that can only be cured by considering appropriate integration contours
which do not go across the cuts in the complex
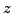 -plane. Other
prescriptions give rise to uncontrolled energy behavior resulting from
the fact that the corresponding integration contours do not close.
-plane. Other
prescriptions give rise to uncontrolled energy behavior resulting from
the fact that the corresponding integration contours do not close.
- As a practical measure that allows avoiding problems related to the
fractional-power density dependence, we propose using the integration
contours which pass near and above the poles. Although such a
prescription requires using rather dense meshes of integration
points, it minimizes the risks of crossing the cuts in the complex
plane. In this way, the ambiguities related to the non-analyticity of
the DFT transition energy are reduced to those corresponding to the
choice of poles included within the integration contour.
- Projected DFT yields questionable results if a pole appears very close to
the integration contour. While such a situation seldom happens
in the ground-state calculations (less than 2% cases are affected),
it frequently occurs in calculations of projected energy
surfaces, such as those in the generator coordinate method (GCM). The
appearance of poles in the vicinity of the contour as a function
of the collective coordinate (e.g., deformation)
gives rise to uncontrolled irregularities and jumps in the results;
in particular, it makes it impossible to define
the PNP potential energy surfaces.
- Pole pathologies appear in a particularly strong way in the
fully self-consistent VAP calculations. In this approach,
transition density poles are not uniquely defined;
moreover, their positions can change during the iteration process leading
to numerical instabilities.
- The analytic structure of the transition energy density becomes
exceedingly
complicated in nuclei with protons and neutrons
paired, thus requiring simultaneous proton and neutron PNP.
Of particular importance in the context of GCM applications
is the extension of the present analysis to non-diagonal matrix elements between
the PNP states.
Some of the problems listed above, in particular those
related to the configuration-mixing DFT method and applications of
the generalized Wick's theorem to DFT, have been recently addressed in a series
of papers [33,34]. In these studies, a practical cure has
been proposed that is based on removing specific spurious components of
the DFT functional that can be associated with self-interaction and self-pairing.
When applied to truncated Hamiltonians,
this practical prescription turns out to be very effective [46].
However, this kind of solution does not remove ambiguities related to
using complicated (e.g., fractional-power) dependence of the energy
density functionals on particle densities. Finding ultimate cures to
the problems discussed in our study will undoubtedly result in
establishing better theoretical constraints on the form of the DFT
energy density functionals for nuclear self-bound systems.
This work was supported in part by the Polish Ministry of
Science; by the Academy of Finland and University of
Jyväskylä within the FIDIPRO programme;
by the U.S. Department of
Energy under Contract Nos. DE-FG02-96ER40963 (University of
Tennessee), DE-AC05-00OR22725 with UT-Battelle, LLC (Oak Ridge
National Laboratory), DE-FG05-87ER40361 (Joint Institute for
Heavy Ion Research), and DE-FC02-07ER41457 (University
of Washington); and by the National Nuclear Security
Administration under the Stewardship Science Academic Alliances
program through DOE Research Grant DE-FG03-03NA00083.



Next: Bibliography
Up: Particle-Number Projection and the
Previous: Deformation energy within the
Jacek Dobaczewski
2007-08-08