


Next: Choice of occupied quasiparticle
Up: Hartree-Fock-Bogoliubov Theory of Polarized
Previous: Introduction
The Quasiparticle Formalism
We begin by recalling basic equations of the quasiparticle formalism,
which historically is attributed to Gor'kov, Bogoliubov, and
de Gennes. While these equations and definitions are
admittedly very
well known,
there are several aspects of the quasiparticle approach that
are seldom discussed; hence, they are worth bringing to the
attention of a wider community. We shall discuss these lesser-known aspects in the
subsections following the general introduction to HFB.
The HFB wave functions are quasiparticle product states.
The quasiparticle annihilation
operators 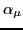 are defined as linear combinations of
particle annihilation and creation operators by the
Bogoliubov transformation,
are defined as linear combinations of
particle annihilation and creation operators by the
Bogoliubov transformation,
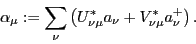 |
(1) |
The matrices 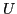 and
and  satisfy the following canonical conditions:
satisfy the following canonical conditions:
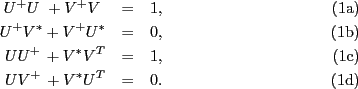 The HFB vacuum
The HFB vacuum 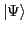 is a zero-quasiparticle state:
is a zero-quasiparticle state:
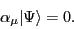 |
(2) |
Complete information about 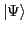 is, in fact, contained
in the generalized density matrix
is, in fact, contained
in the generalized density matrix 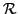 ,
,
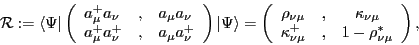 |
(3) |
which in terms of matrices 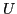 and
and  reads:
reads:
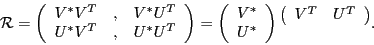 |
(4) |
The variational principle implies
that the self-consistent density matrix 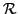 commutes with
the quasiparticle Hamiltonian
commutes with
the quasiparticle Hamiltonian
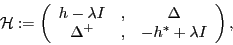 |
(5) |
where 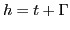 is the single-particle Hamiltonian and
is the single-particle Hamiltonian and
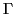 and
and 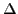 are particle-hole and particle-particle
mean-fields, respectively. The HFB equations can be written
in a matrix form:
are particle-hole and particle-particle
mean-fields, respectively. The HFB equations can be written
in a matrix form:
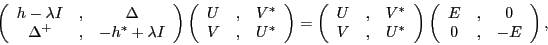 |
(6) |
where 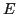 is a diagonal matrix of quasiparticle energies
is a diagonal matrix of quasiparticle energies 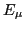 .
Columns of
eigenvectors,
.
Columns of
eigenvectors,
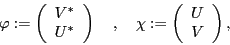 |
(7) |
are called occupied and empty quasiparticle states, respectively,
because they are eigenvectors of the projective matrix 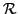 with eigenvalues 1 and 0.
with eigenvalues 1 and 0.
Subsections



Next: Choice of occupied quasiparticle
Up: Hartree-Fock-Bogoliubov Theory of Polarized
Previous: Introduction
Jacek Dobaczewski
2009-04-13
![]() are defined as linear combinations of
particle annihilation and creation operators by the
Bogoliubov transformation,
are defined as linear combinations of
particle annihilation and creation operators by the
Bogoliubov transformation,
 The HFB vacuum
The HFB vacuum