


Next: Hartree-Fock-Bogoliubov theory
Up: Hartree-Fock-Bogoliubov solution of the
Previous: Introduction
Basics of pairing correlations
In quantum mechanics of finite many-fermion systems, pairing
correlations are best described in terms of number
operators
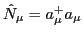 , where
, where 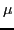 represents any
suitable set of single-particle (s.p.) quantum numbers. Thus we may have,
e.g.,
represents any
suitable set of single-particle (s.p.) quantum numbers. Thus we may have,
e.g.,
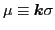 for plane waves of spin-
for plane waves of spin-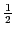 particles (electrons);
particles (electrons);
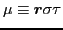 for spin-
for spin-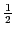 and
isospin-
and
isospin-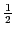 nucleons localized in space at position
nucleons localized in space at position  ; and
; and
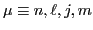 for fermions moving in a spherical potential
well. Since
for fermions moving in a spherical potential
well. Since
 , the number operators are
projective; hence, one can - at least in principle - devise
an experiment that would project any quantum many-fermion state
, the number operators are
projective; hence, one can - at least in principle - devise
an experiment that would project any quantum many-fermion state
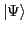 into its component with exactly one fermion occupying
state
into its component with exactly one fermion occupying
state 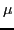 . As the rules of quantum mechanics stipulate, any such
individual measurement can only give 0 or 1 (these are the
eigenvalues of
. As the rules of quantum mechanics stipulate, any such
individual measurement can only give 0 or 1 (these are the
eigenvalues of 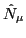 ), whereas performing such measurements
many times, one could experimentally determine the
occupation probabilities
), whereas performing such measurements
many times, one could experimentally determine the
occupation probabilities
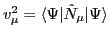 .
.
Along such lines, we can devise an
experiment that would determine the simultaneous presence of two
fermions in different orthogonal s.p. states 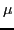 and
and
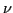 . Since the corresponding number operators
. Since the corresponding number operators 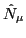 and
and
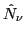 commute, one can legitimately ask quantum
mechanical questions about one-particle occupation probabilities
commute, one can legitimately ask quantum
mechanical questions about one-particle occupation probabilities
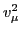 and
and 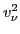 , as well as about the two-particle occupation.
The latter one reflects the simultaneous presence of two fermions in state
, as well as about the two-particle occupation.
The latter one reflects the simultaneous presence of two fermions in state
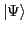 :
:
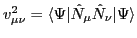 .
In this way, one can experimentally determine the pairing correlation
between states
.
In this way, one can experimentally determine the pairing correlation
between states 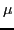 and
and 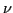 as the excess probability
as the excess probability
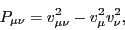 |
(1) |
of finding two fermions simultaneously over that of finding them in
independent, or sequential, measurements. Such a definition of
pairing is independent of its coherence, collectivity, nature of
quasiparticles, symmetry breaking, thermodynamic limit, or many other
notions that are often associated with the phenomenon of pairing. In
terms of occupations, pairing can be viewed as a measurable
property of any quantum many-fermion state.
Obviously, no pairing correlations are present in a quantum state
that is an eigenstate of 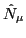 or
or 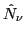 ,
such as the Slater determinant.
The beauty of the BCS ansatz is in providing us
with a model
,
such as the Slater determinant.
The beauty of the BCS ansatz is in providing us
with a model 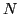 -fermion state, in which pairing correlations are
explicitly incorporated:
-fermion state, in which pairing correlations are
explicitly incorporated:
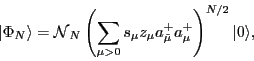 |
(2) |
where the summation 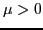 runs over the representatives of pairs
(
runs over the representatives of pairs
(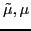 ) of s.p. states (that is, any one state of
the pair is included in the sum, but not both),
) of s.p. states (that is, any one state of
the pair is included in the sum, but not both),
 are real positive numbers,
are real positive numbers,
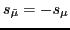 are arbitrary
complex phase factors, and
are arbitrary
complex phase factors, and 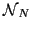 is the overall normalization
factor.
is the overall normalization
factor.
It now becomes a matter of technical convenience to employ
a particle-number mixed state,
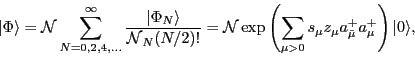 |
(3) |
in which the pairing correlations (1) are:
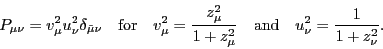 |
(4) |
In terms of the s.p. occupations, the state  assumes
the standard BCS form:
assumes
the standard BCS form:
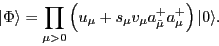 |
(5) |
In this many-fermion state, the s.p. states 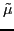 and
and 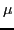 are paired, that is,
are paired, that is,  can be viewed as a
pair-condensate. For
can be viewed as a
pair-condensate. For 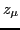 =1, the pairing correlation
=1, the pairing correlation
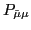 (1) equals 1/4; in fact, in this state,
it is twice more likely to find a pair of fermions
(
(1) equals 1/4; in fact, in this state,
it is twice more likely to find a pair of fermions
(
 =1/2) than to find these two fermions
independently (
=1/2) than to find these two fermions
independently (
 =1/4). For particle-number
conserving states (2), the occupation numbers can be
calculated numerically; qualitatively the results are fairly similar,
especially for large numbers of particles.
=1/4). For particle-number
conserving states (2), the occupation numbers can be
calculated numerically; qualitatively the results are fairly similar,
especially for large numbers of particles.
At this point, we note that the most general pair-condensate state (3)
has the form of the Thouless state,
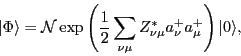 |
(6) |
in which pairs (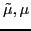 ) do not appear explicitly. However,
there always exists a unitary transformation
) do not appear explicitly. However,
there always exists a unitary transformation 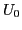 of the antisymmetric
matrix
of the antisymmetric
matrix 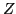 that brings it to the canonical form
that brings it to the canonical form
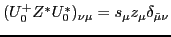 (the
Bloch-Messiah-Zumino theorem[9,10]). Therefore,
pairs are present in any arbitrary Thouless state (the so-called
canonical pairs), and they can be made explicitly visible by a simple
basis transformation.
(the
Bloch-Messiah-Zumino theorem[9,10]). Therefore,
pairs are present in any arbitrary Thouless state (the so-called
canonical pairs), and they can be made explicitly visible by a simple
basis transformation.
The canonical pairs exist independently of any
symmetry of the Thouless state. In the particular case of a
time-reversal-symmetric state,
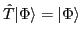 ,
they can be associated with the time-reversed s.p. states,
,
they can be associated with the time-reversed s.p. states,
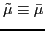 . The ground-states of even-even nuclei
can be described in this manner. However, the appearance of pairing
phase does not hinge on this particular symmetry - states in
rotating nuclei (in which time-reversal symmetry is manifestly
broken) can also be paired. In this latter case the canonical states
are less useful, because they cannot be directly associated with the
eigenstates of the HFB Hamiltonian.
. The ground-states of even-even nuclei
can be described in this manner. However, the appearance of pairing
phase does not hinge on this particular symmetry - states in
rotating nuclei (in which time-reversal symmetry is manifestly
broken) can also be paired. In this latter case the canonical states
are less useful, because they cannot be directly associated with the
eigenstates of the HFB Hamiltonian.



Next: Hartree-Fock-Bogoliubov theory
Up: Hartree-Fock-Bogoliubov solution of the
Previous: Introduction
Jacek Dobaczewski
2012-07-17
![]() and
and
![]() . Since the corresponding number operators
. Since the corresponding number operators ![]() and
and
![]() commute, one can legitimately ask quantum
mechanical questions about one-particle occupation probabilities
commute, one can legitimately ask quantum
mechanical questions about one-particle occupation probabilities
![]() and
and ![]() , as well as about the two-particle occupation.
The latter one reflects the simultaneous presence of two fermions in state
, as well as about the two-particle occupation.
The latter one reflects the simultaneous presence of two fermions in state
![]() :
:
![]() .
In this way, one can experimentally determine the pairing correlation
between states
.
In this way, one can experimentally determine the pairing correlation
between states ![]() and
and ![]() as the excess probability
as the excess probability
![]() or
or ![]() ,
such as the Slater determinant.
The beauty of the BCS ansatz is in providing us
with a model
,
such as the Slater determinant.
The beauty of the BCS ansatz is in providing us
with a model ![]() -fermion state, in which pairing correlations are
explicitly incorporated:
-fermion state, in which pairing correlations are
explicitly incorporated:
![]() ,
they can be associated with the time-reversed s.p. states,
,
they can be associated with the time-reversed s.p. states,
![]() . The ground-states of even-even nuclei
can be described in this manner. However, the appearance of pairing
phase does not hinge on this particular symmetry - states in
rotating nuclei (in which time-reversal symmetry is manifestly
broken) can also be paired. In this latter case the canonical states
are less useful, because they cannot be directly associated with the
eigenstates of the HFB Hamiltonian.
. The ground-states of even-even nuclei
can be described in this manner. However, the appearance of pairing
phase does not hinge on this particular symmetry - states in
rotating nuclei (in which time-reversal symmetry is manifestly
broken) can also be paired. In this latter case the canonical states
are less useful, because they cannot be directly associated with the
eigenstates of the HFB Hamiltonian.