Next: Multipole, surface multipole, and
Up: Modifications introduced in version
Previous: Breaking of all the
Tilted-axis cranking
The tilted-axis cranking is realized by using in the energy
functional the extended cranking term, cf. Eq. (I-23), which now reads
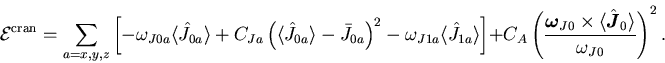 |
(1) |
The first two terms under the sum are simple generalizations of the
standard linear and quadratic spin constraints to three dimensions.
Different stiffness constants 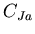 are allowed in three Cartesian
directions
are allowed in three Cartesian
directions  =
=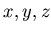 . These standard constraints act on the
standard isoscalar (total) average angular momentum vector,
. These standard constraints act on the
standard isoscalar (total) average angular momentum vector,
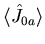 =
=
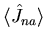 +
+
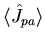 , which is a sum of the neutron (
, which is a sum of the neutron (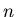 ) and proton (
) and proton (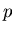 )
component. The third term under the sum constitutes a linear
constraint on the isovector average angular momentum vector,
)
component. The third term under the sum constitutes a linear
constraint on the isovector average angular momentum vector,
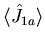 =
=
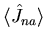
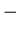
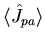 . It is introduced to
facilitate the fixing of separate neutron and proton angular momentum
vectors, which can be essential when trying to localize, e.g., the
shears configurations. Of course, after a proper configuration is
found, the final constraints should involve only the isoscalar
component of the angular momentum vector. The last term in Eq. (1) constraints to zero the angle between the angular
frequency and angular momentum vectors. Since in the self-consistent
solutions this angle must be equal to zero [7], the last
constraint helps to reach the self-consistent solution faster.
However, since it is build upon the angular frequency vector
pertaining to the linear isoscalar constraint, it cannot be used
neither in conjunction with the quadratic nor with the isovector
constraint.
. It is introduced to
facilitate the fixing of separate neutron and proton angular momentum
vectors, which can be essential when trying to localize, e.g., the
shears configurations. Of course, after a proper configuration is
found, the final constraints should involve only the isoscalar
component of the angular momentum vector. The last term in Eq. (1) constraints to zero the angle between the angular
frequency and angular momentum vectors. Since in the self-consistent
solutions this angle must be equal to zero [7], the last
constraint helps to reach the self-consistent solution faster.
However, since it is build upon the angular frequency vector
pertaining to the linear isoscalar constraint, it cannot be used
neither in conjunction with the quadratic nor with the isovector
constraint.
In practical calculations, it turns out that the angle between the
angular frequency and angular momentum converges to zero extremely
slowly. This is so because for an angular frequency vector fixed in
space, the whole nucleus must turn in space in order to align its
angular momentum with the angular frequency. Therefore, a much faster
procedure is to proceed in an opposite way, i.e., in each iteration
to force the angular frequency to be aligned or anti-aligned with the
current angular momentum vector (see switch IMOVAX in Sec. 3.5). This is a purely heuristic procedure, because it does
not correspond to a minimization of any given Routhian. However, once
the self-consistent solution is found (the angular frequency and
angular momentum vectors become parallel or antiparallel) it is the
Routhian for the final angular frequency which has taken the minimum
value.



Next: Multipole, surface multipole, and
Up: Modifications introduced in version
Previous: Breaking of all the
Jacek Dobaczewski
2004-01-06