Volume and surface components of the energy density

Without any detailed microscopic knowledge of the nuclear effective interactions, we can rely on general properties of saturating fermion systems to assume that the total energy ${\cal E}$ of a nucleus is an integral of a local energy density ${\cal H}(\mbox{{\boldmath {$r$ }}})$. Such a conjecture is a basis for the so-called Local Density Approximation (LDA) that has been extensively used in the context of atomic and molecular physics. In nuclear physics LDA has been employed in the form of the Skyrme-HF approximation, in which the total energy ${\cal E}$is given as

$${\cal E} = {\cal E}_{\mbox{\scriptsize {kin}}} + {\cal E}_... ...{\scriptsize {Coul}}} + {\cal E}_{\mbox{\scriptsize {pair}}},$$ (1)

or equivalently

 

$${\cal E} = \int d^3\mbox{{\boldmath {$r$ }}} \!\!\Big[\!\! {\cal H}_{\mbox{\scriptsize {kin}}} (\mbox{{\boldmath {$r$ }}}) +... ...ath {$r$ }}}) + {\cal H}_{\mbox{\scriptsize {S-O}}} (\mbox{{\boldmath {$r$ }}})$$

$${\cal H}_{\mbox{\scriptsize {Coul}}} (\mbox{{\boldmath {$r$ }}}) + {\cal H}_{\mbox{\scriptsize {pair}}} (\mbox{{\boldmath {$r$ }}}) \Big].$$ + (2)

The kinetic ( ${\cal H}_{\mbox{\scriptsize {kin}}}$), Skyrme ( ${\cal H}_{\mbox{\scriptsize {Skyrme}}}$), spin-orbit ( ${\cal H}_{\mbox{\scriptsize {S-O}}}$), Coulomb ( ${\cal H}_{\mbox{\scriptsize {Coul}}}$), and pairing ( ${\cal H}_{\mbox{\scriptsize {pair}}}$) densities are functions of several local densities:

   

$${\cal H}_{\mbox{\scriptsize {kin}}} (\mbox{{\boldmath {$r$ }}}) \frac{\hbar^2}{2m}\left(1-\frac{1}{A}\right) \tau_0 ,$$ = (3)

$$\!\!\!{\cal H}_{\mbox{\scriptsize {Skyrme}}}(\mbox{{\boldmath {$r$ }}}) {\displaystyle\sum_{t=0,1}}\Big[ C_t^{\rho}(\rho_0) \rho_t^2 \!+\!C_t^{\Delta\rho} \rho_t\Delta\rho_t \!+\!C_t^{\tau} \rho_t\tau_t\Big],$$ = (4)

$${\cal H}_{\mbox{\scriptsize {S-O}}} (\mbox{{\boldmath {$r$ }}}) {\displaystyle\sum_{t=0,1}}\Big( C_t^{\nabla J} \rho_t\mbox{{\boldmath {$\nabla$ }}}\cdot\mbox{{\boldmath {$J$ }}}_t\Big) ,$$ = (5)

$${\cal H}_{\mbox{\scriptsize {Coul}}} (\mbox{{\boldmath {$r$ }}}) V_{\mbox{\scriptsize {Coul}}} (\rho_p) -\frac{3e^2}{4}\left(\frac{3}{\pi}\right)^{1/3} \rho_p^{4/3} ,$$ = (6)

$${\cal H}_{\mbox{\scriptsize {pair}}} (\mbox{{\boldmath {$r$ }}}) \frac{1}{4}f_{\mbox{\scriptsize {pair}}}(\rho_0) {\displaystyle\sum_{t=0,1}}\kappa_t^2 ,$$ = (7)

where, e.g., $\rho_0$=$\rho_n$+$\rho_p$ and $\rho_1$=$\rho_n$-$\rho_p$are the isoscalar and isovector particle densities, respectively, and $\rho_n$ and $\rho_p$are the corresponding neutron and proton densities. For complete definitions of other densities and coupling constants appearing in expressions (3)-(7), the reader is referred, e.g., to Refs. [1,2]. (In Eq. (4) we have omitted the term depending on the tensor spin-current density because below we use only the Skyrme force in which this particular term was neglected.)

In the lower panel of Fig. 1 the above five energy densities are plotted for ¹²⁰Sn, together with their sum ${\cal H}_{\mbox{\scriptsize {tot}}}$. Calculations were performed for the Skyrme interaction SLy4 [3] and the volume pairing force. (See Ref. [4] for details of the calculations.) The presented results are very generic, and identical qualitative results are obtained for any other nucleus or interaction.

  

Figure 1: Total energy density ${\cal H}_{\mbox{\scriptsize {tot}}}$ (full circles) calculated within the Skyrme-HF-SLy4 method for ¹²⁰Sn, and plotted together with its five components given by decompositions defined in Eqs. (2) (lower panel) and (9) (upper panel).

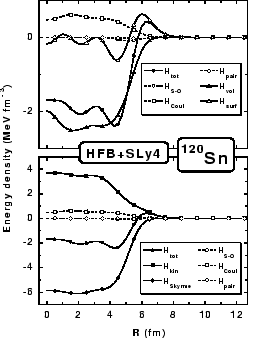Only three out of five components significantly contribute to the total energy density, namely, the kinetic, Skyrme, and Coulomb densities. The remaining two have, of course, a decisive influence on detailed properties of nuclei, however, they are almost invisible in the scale of Fig. 1, and for the sake of the following discussion can be safely put aside. We can also see that the kinetic energy density dominates in the surface region; indeed, both the Skyrme and Coulomb terms simultaneously go to zero at a distance that is by about 1fm smaller than the place where the kinetic energy vanishes.

Therefore, in order to analyze the energy relations at the nuclear surface, it is essential to consider surface properties of the kinetic energy density. Semiclassical methods are not appropriate to separate the volume and surface contributions to ${\cal H}_{\mbox{\scriptsize {kin}}}$, because such approaches are not valid beyond the classical turning point. Therefore, one is often fitting the volume and surface terms to reproduce the microscopic values of ${\cal H}_{\mbox{\scriptsize {kin}}}$ (see, e.g., [5]). From such analyses it turns out that the volume contribution is very well described by the nuclear-matter expression

$$\tau^{\mbox{\scriptsize {nm}}}_{n,p} = \frac{\pi^{4/3}}{5}(3\rho_{n,p})^{5/3}.$$ (8)

Hence, in the present study we simply consider the remaining part of the kinetic energy density to be the surface contribution.

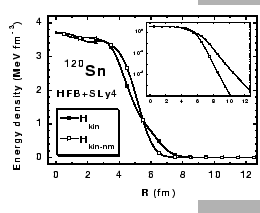
In Fig. 2 we compare the microscopic kinetic energy density ${\cal H}_{\mbox{\scriptsize {kin}}}$, Eq. (3), with the corresponding nuclear-matter (volume) contribution ${\cal H}_{\mbox{\scriptsize {kin-nm}}}$obtained by replacing $\tau_0=\tau_n+\tau_p$ with $\tau^{\mbox{\scriptsize {nm}}}_0=\tau^{\mbox{\scriptsize {nm}}}_n+\tau^{\mbox{\scriptsize {nm}}}_p$. The difference between the two curves gives our surface contribution to the kinetic energy density.

  

Figure 2: Microscopic kinetic energy density ${\cal H}_{\mbox{\scriptsize {kin}}}$(full circles) compared to the nuclear-matter (volume) approximation ${\cal H}_{\mbox{\scriptsize {kin-nm}}}$ (open circles).

Based on these arguments, we can now rearrange terms in ${\cal E}$ in such a way as to single out the volume and surface contributions:

 

$${\cal E} = \int d^3\mbox{{\boldmath {$r$ }}} \!\!\Big[\!\! {\cal H}_{\mbox{\scriptsize {vol}}} (\mbox{{\boldmath {$r$ }}}) +... ...ath {$r$ }}}) + {\cal H}_{\mbox{\scriptsize {S-O}}} (\mbox{{\boldmath {$r$ }}})$$

$${\cal H}_{\mbox{\scriptsize {Coul}}} (\mbox{{\boldmath {$r$ }}}) + {\cal H}_{\mbox{\scriptsize {pair}}} (\mbox{{\boldmath {$r$ }}}) \Big],$$ + (9)

where

  

$${\cal H}_{\mbox{\scriptsize {vol}}} (\mbox{{\boldmath {$r$ }}}) \frac{\hbar^2}{2m}\left(1-\frac{1}{A}\right) \tau^{\mbox{\scriptsize {nm}}}_0$$ =

$${\displaystyle\sum_{t=0,1}}\Big[ C_t^{\rho}(\rho_0) \rho_t^2 + C_t^{\tau} \rho_t\tau^{\mbox{\scriptsize {nm}}}_t\Big] ,$$ + (10)

$${\cal H}_{\mbox{\scriptsize {surf}}} (\mbox{{\boldmath {$r$ }}}) \frac{\hbar^2}{2m}\left(1-\frac{1}{A}\right) \Big(\tau_0 -\tau^{\mbox{\scriptsize {nm}}}_0\Big)$$ =

$${\displaystyle\sum_{t=0,1}}\Big[ C_t^{\Delta\rho} \rho_t\Delta\rho_t + C_t^{\tau} \rho_t\Big(\tau_t -\tau^{\mbox{\scriptsize {nm}}}_t\Big)\Big],$$ + (11)

and ${\cal H}_{\mbox{\scriptsize {vol}}} (\mbox{{\boldmath {$r$ }}}) + {\cal H}_{\m... ... {$r$ }}}) + {\cal H}_{\mbox{\scriptsize {Skyrme}}}(\mbox{{\boldmath {$r$ }}})$. In the Skyrme energy density (4) the effective-mass terms have been separated into the volume and surface parts according to the prescription defined above for the bare-mass terms.

In Fig. 1 (upper panel) are plotted the volume (10) and surface (11) contributions to the ¹²⁰Sn total density energy. It is clear that in the surface region of this nucleus (5-7fm) these two contributions are of a similar magnitude and opposite sign. Therefore, the nuclear surface cannot simply be regarded as a layer of nuclear matter at low density. In this zone the gradient terms (absent in the nuclear matter) are as important in defining the energy relations as those depending on the local density.

This observation exemplifies the difficulties in extracting the pairing properties of finite nuclei from the nuclear-matter calculations. In particular, the nuclear-matter pairing intensity, calculated at densities below the saturation point, need not be the same as the analogous intensity at the surface of a nucleus. The nuclear-matter and neutron-matter calculations of the pairing gap (see Ref. [6] for a review) performed at various densities by using very advanced and sophisticated methods, as well the best bare NN forces, can therefore be, at most, considered as weak indications of what might be the actual situation in nuclei. In this respect, calculations in semi-infinite matter that recently became available [7] may provide much more reliable information.