


Next: Time-reversal symmetry
Up: Conserved symmetries
Previous: Conserved symmetries
Proton-neutron symmetry
The standard case of no proton-neutron mixing can be described
by the conserved proton-neutron symmetry given by
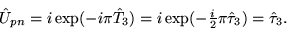 |
(214) |
In other words, the iso-3 signature (multiplied by 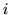 ) is then the
conserved symmetry. Note that conservation of projection of the
isospin on the third axis (the charge conservation) would require
that the iso-3 rotation about an arbitrary angle be conserved, while
the iso-3 signature corresponds only to rotation about
) is then the
conserved symmetry. Note that conservation of projection of the
isospin on the third axis (the charge conservation) would require
that the iso-3 rotation about an arbitrary angle be conserved, while
the iso-3 signature corresponds only to rotation about 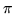 . Within
the HFB approach, the charge symmetry is broken in the same way as is the
particle number symmetry.
. Within
the HFB approach, the charge symmetry is broken in the same way as is the
particle number symmetry.
Since the 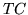 -transformed symmetry operator reads
-transformed symmetry operator reads
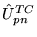 =
= , we obtain from Eq. (213)
that
, we obtain from Eq. (213)
that
and analogous properties hold for the mean-field Hamiltonians,
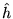 and
and
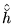 , respectively. It is then clear that
without the proton-neutron mixing the p-h density matrices
and Hamiltonians have only the
, respectively. It is then clear that
without the proton-neutron mixing the p-h density matrices
and Hamiltonians have only the 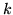 =0 and 3 isospin components, while
the p-p ones have (in the ``breve'' representation) only the
=0 and 3 isospin components, while
the p-p ones have (in the ``breve'' representation) only the
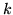 =1 and 2 isospin components, cf. Eqs. (58) and
(60).
=1 and 2 isospin components, cf. Eqs. (58) and
(60).



Next: Time-reversal symmetry
Up: Conserved symmetries
Previous: Conserved symmetries
Jacek Dobaczewski
2004-01-03
![]() -transformed symmetry operator reads
-transformed symmetry operator reads
![]() =
=![]() , we obtain from Eq. (213)
that
, we obtain from Eq. (213)
that