


Next: Two-dimensional rotational symmetry SO
Up: SPATIAL SYMMETRIES OF THE
Previous: Rotational and mirror symmetry
Axial symmetry
Let us suppose that rotations and mirror rotations
around one axis (say 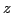 -axis) are SCSs. This symmetry
group will be denoted as O
-axis) are SCSs. This symmetry
group will be denoted as O
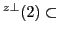 O(3). It is the direct
product O
O(3). It is the direct
product O
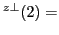 S
S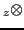 SO
SO
 of the
SO
of the
SO
 group of rotations around the
group of rotations around the 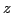 -axis and the
two-element group S
-axis and the
two-element group S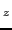 consisting of the reflection in the plane
perpendicular to the symmetry axis and the identity. To investigate
the axial symmetry, it is convenient to decompose the position vector
consisting of the reflection in the plane
perpendicular to the symmetry axis and the identity. To investigate
the axial symmetry, it is convenient to decompose the position vector
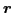 into the components parallel and perpendicular to the
symmetry axis:
into the components parallel and perpendicular to the
symmetry axis:
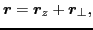 |
(29) |
which have different transformation properties under the
O
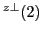 transformations. The component
transformations. The component
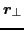 is
a SO
is
a SO
 vector whereas
vector whereas  is not affected by the
SO
is not affected by the
SO
 rotations; hence, it is invariant under SO
rotations; hence, it is invariant under SO
 .
On the other hand,
.
On the other hand,  changes
its sign under the reflection S
changes
its sign under the reflection S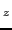 , while
, while
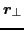 is
S
is
S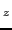 -invariant.
-invariant.
Subsections
Jacek Dobaczewski
2010-01-30