


Next: Two-dimensional rotational and mirror
Up: Axial symmetry
Previous: Axial symmetry
Two-dimensional rotational symmetry SO

If the density matrices
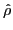 and
and
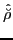 possess the
SO
possess the
SO
 symmetry, it follows from Eqs. (5) and
(6) that all densities of type
symmetry, it follows from Eqs. (5) and
(6) that all densities of type  should be
SO
should be
SO
 scalars.
Now it is convenient to decompose densities of type
scalars.
Now it is convenient to decompose densities of type 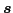 into components perpendicular and parallel to the symmetry axis:
into components perpendicular and parallel to the symmetry axis:
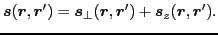 |
(30) |
The perpendicular components
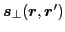 are SO
are SO
 vectors and the parallel components
vectors and the parallel components
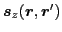 are
SO
are
SO
 invariants.
invariants.
The following SO
 scalars can be constructed from the perpendicular and parallel components
of the
position vectors
scalars can be constructed from the perpendicular and parallel components
of the
position vectors 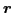 and
and 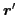 :
: 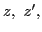 the
the 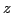 -coordinates of
-coordinates of  and
and 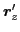 , respectively,
, respectively,
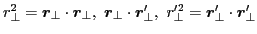 ,
and
,
and
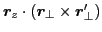 and
and
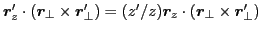 .
As we see the two latter scalars are dependent on each other because
.
As we see the two latter scalars are dependent on each other because
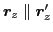 .
The square
.
The square
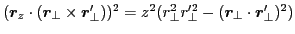 is
dependent on other scalars, namely on
is
dependent on other scalars, namely on
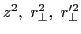 and
and
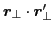 .
Hence, the
nonlocal scalar densities have the form
.
Hence, the
nonlocal scalar densities have the form
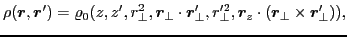 |
(31) |
where 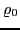 is an arbitrary scalar function, linear in the last argument. There are three
vectors invariant under SO
is an arbitrary scalar function, linear in the last argument. There are three
vectors invariant under SO
 and parallel to the symmetry
axis, namely
and parallel to the symmetry
axis, namely  ,
, 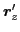 , and
, and
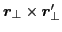 . Consequently, the z-component
of
. Consequently, the z-component
of
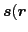 ,
, 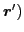 is of the form
is of the form
where 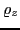 ,
,
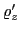 , and
, and 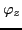 are scalar functions.
There exist four independent SO
are scalar functions.
There exist four independent SO
 vectors that lie in the
plane perpendicular to the symmetry axis:
vectors that lie in the
plane perpendicular to the symmetry axis:
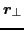 ,
,
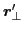 ,
,
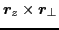 and
and
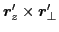 . Hence, the SO
. Hence, the SO
 vector component of the nonlocal spin density takes the
form:
vector component of the nonlocal spin density takes the
form:
with
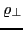 ,
,
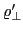 ,
,
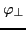 , and
, and
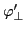 being scalar functions.
General forms of the local zero-order densities are obtained
from Eqs. (31), (32), and
(33) by putting
being scalar functions.
General forms of the local zero-order densities are obtained
from Eqs. (31), (32), and
(33) by putting
 and
and
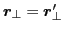 . This gives:
. This gives:
where 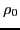 ,
, 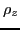 ,
,
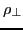 , and
, and
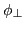 are
arbitrary scalar functions of
are
arbitrary scalar functions of 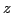 and
and
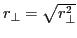 . The general form of the remaining
scalar densities
. The general form of the remaining
scalar densities
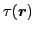 and
and 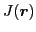 is the same as that of
Eq. (34).
is the same as that of
Eq. (34).
The components of the differential operator,
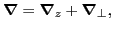 |
(37) |
have the same transformation properties under SO
 rotations as the corresponding components of the position
vector (29). Hence, the densities
rotations as the corresponding components of the position
vector (29). Hence, the densities
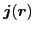 ,
,
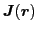 ,
,
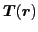 , and
, and
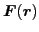 have the components parallel to the
have the components parallel to the 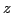 -axis that are invariant
under SO
-axis that are invariant
under SO
 and the SO
and the SO
 vector components
that are
perpendicular to the symmetry axis. Therefore, they all take general forms
given by Eqs. (35) and
(36).
vector components
that are
perpendicular to the symmetry axis. Therefore, they all take general forms
given by Eqs. (35) and
(36).
It follows from the definitions (12),
(15), and (37) that the components
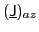 (
(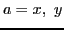 ) of the symmetric
traceless spin-current density form the SO
) of the symmetric
traceless spin-current density form the SO
 vector while
the components
vector while
the components
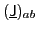 (
(
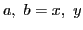 ) form
the SO
) form
the SO
 symmetric traceless tensor. The following four
symmetric traceless tensors can be formed with vectors
symmetric traceless tensor. The following four
symmetric traceless tensors can be formed with vectors  and
and
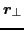 :
:
where
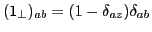 (
(
 ) is the unit tensor in the plane perpendicular to the
symmetry axis. Two tensors (38) and
(39) transform under SO
) is the unit tensor in the plane perpendicular to the
symmetry axis. Two tensors (38) and
(39) transform under SO
 like
vectors perpendicular to the
like
vectors perpendicular to the 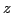 -axis. The tensors
(40) and (41) are the SO
-axis. The tensors
(40) and (41) are the SO
 tensors. Consequently, the general form of the symmetric traceless spin-current
density is:
tensors. Consequently, the general form of the symmetric traceless spin-current
density is:
where
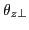 ,
,
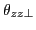 ,
,
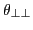 ,
and
,
and
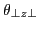 are scalar functions. We note in passing
that in the case of the axial symmetry, all local densities
formally look like the spherical symmetric nonlocal fields
(20), (21), and (22) with
are scalar functions. We note in passing
that in the case of the axial symmetry, all local densities
formally look like the spherical symmetric nonlocal fields
(20), (21), and (22) with
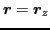 and
and
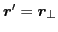 , provided that
, provided that
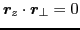 .
.



Next: Two-dimensional rotational and mirror
Up: Axial symmetry
Previous: Axial symmetry
Jacek Dobaczewski
2010-01-30
![]() and
and
![]() possess the
SO
possess the
SO
![]() symmetry, it follows from Eqs. (5) and
(6) that all densities of type
symmetry, it follows from Eqs. (5) and
(6) that all densities of type ![]() should be
SO
should be
SO
![]() scalars.
Now it is convenient to decompose densities of type
scalars.
Now it is convenient to decompose densities of type ![]() into components perpendicular and parallel to the symmetry axis:
into components perpendicular and parallel to the symmetry axis:
![]() scalars can be constructed from the perpendicular and parallel components
of the
position vectors
scalars can be constructed from the perpendicular and parallel components
of the
position vectors ![]() and
and ![]() :
: ![]() the
the ![]() -coordinates of
-coordinates of ![]() and
and ![]() , respectively,
, respectively,
![]() ,
and
,
and
![]() and
and
![]() .
As we see the two latter scalars are dependent on each other because
.
As we see the two latter scalars are dependent on each other because
![]() .
The square
.
The square
![]() is
dependent on other scalars, namely on
is
dependent on other scalars, namely on
![]() and
and
![]() .
Hence, the
nonlocal scalar densities have the form
.
Hence, the
nonlocal scalar densities have the form
![]() (
(![]() ) of the symmetric
traceless spin-current density form the SO
) of the symmetric
traceless spin-current density form the SO
![]() vector while
the components
vector while
the components
![]() (
(
![]() ) form
the SO
) form
the SO
![]() symmetric traceless tensor. The following four
symmetric traceless tensors can be formed with vectors
symmetric traceless tensor. The following four
symmetric traceless tensors can be formed with vectors ![]() and
and
![]() :
: