Next: Quadratic regularization scheme Up: Regularization scheme Previous: Standard MR DFT scheme
The LR scheme applies, for example, to the density-independent SV interaction in its original formulation [18], that is, without the EDF tensor terms. It is also applicable to the density-dependent SIII functional [18]. The reason is that the density-dependence of this latter force does not lead, for a given type of particles, to a higher power of density. The third example, where the LR should be sufficient, is the Coulomb exchange treated in the so called Slater approximation [25], because in this approximation the exchange Coulomb transition matrix element behaves as
| (19) |
The overlap is always regular and, therefore, it can be expanded in terms of the Wigner ![]() -functions as,
-functions as,
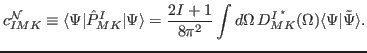 |
(21) |
Inserting (23) to (22), and requesting that Eq. (17) holds at ![]() ,
gives rise to a set of linear equations for regularized matrix elements
,
gives rise to a set of linear equations for regularized matrix elements
![]() :
:
We note here that the regularization procedure can be applied separately to all terms of the EDF, that is, terms that correspond to interactions can be treated within the standard AMP method, and only those which do not, should be treated within the regularization scheme.
The expansion of Slater determinant
![]() in terms of the AMP states
reads [6],
in terms of the AMP states
reads [6],
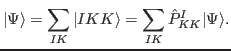 |
(26) |
More precisely, we focus our attention on investigating stability of
these two quantities in function of the highest angular momentum
![]() included in the calculations, and we present them
versus
included in the calculations, and we present them
versus
![]() . The same value of
. The same value of
![]() is
consistently used to define both summation ranges in
Eqs. (24) and (25). Note that in Eq. (25),
the range of summation should be higher than the natural cutoff
dictated by the highest meaningful AMP components in the mean-field
wave function, which are given by the values of amplitudes
is
consistently used to define both summation ranges in
Eqs. (24) and (25). Note that in Eq. (25),
the range of summation should be higher than the natural cutoff
dictated by the highest meaningful AMP components in the mean-field
wave function, which are given by the values of amplitudes
![]() . With increasing values of
. With increasing values of
![]() , the residuals of sum rules (27) and
(28), should converge to zero.
, the residuals of sum rules (27) and
(28), should converge to zero.
All calculations were performed using the unrestricted-symmetry
solver HFODD [26,27]. We employed the Gauss-Chebyshev
quadratures to integrate over the ![]() and
and ![]() Euler angles
and the Gauss-Legendre quadrature to integrate over the
Euler angles
and the Gauss-Legendre quadrature to integrate over the ![]() Euler
angle. To achieve a sufficient accuracy, for each Euler angle we
used a large number of mesh points equal
Euler
angle. To achieve a sufficient accuracy, for each Euler angle we
used a large number of mesh points equal
![]() .
.
The examples
presented below pertain to odd-odd nucleus ![]() Al, and to the so
called anti-aligned mean-field configuration, which is relevant in
the context of the superallowed Fermi
Al, and to the so
called anti-aligned mean-field configuration, which is relevant in
the context of the superallowed Fermi ![]() -decay [19].
The most demanding task was to calculate the auxiliary integrals
-decay [19].
The most demanding task was to calculate the auxiliary integrals
![]() , Eq. (13). Since we were interested
in comparing the standard and regularized calculations, we decided
to use a relatively small configuration space, consisting of only
, Eq. (13). Since we were interested
in comparing the standard and regularized calculations, we decided
to use a relatively small configuration space, consisting of only
![]() spherical harmonic-oscillator shells. Such small space
suppresses high angular-momentum components in the reference Slater
determinant. Unless explicitly stated, in all calculations, in both
direct and exchange channels the Coulomb interaction was treated
exactly.
spherical harmonic-oscillator shells. Such small space
suppresses high angular-momentum components in the reference Slater
determinant. Unless explicitly stated, in all calculations, in both
direct and exchange channels the Coulomb interaction was treated
exactly.
![\includegraphics[width=0.5\columnwidth]{Reg3aFig1.eps}](img96.png) |
It is instructive to begin the discussion by showing results for
![]() and Skyrme-energy sum-rule residuals obtained for the
SV
and Skyrme-energy sum-rule residuals obtained for the
SV![]() Skyrme force. Such a calculation tests the numerical
implementation of the method, and can be regarded as a proof of
principle of the LR scheme. The reason is, as already mentioned, that
the SV
Skyrme force. Such a calculation tests the numerical
implementation of the method, and can be regarded as a proof of
principle of the LR scheme. The reason is, as already mentioned, that
the SV![]() is a true interaction and, therefore, both
standard AMP method and LR method should give exactly the same values
of both indicators. As can be seen in Fig. 1, this is
indeed the case. It turns out that for
is a true interaction and, therefore, both
standard AMP method and LR method should give exactly the same values
of both indicators. As can be seen in Fig. 1, this is
indeed the case. It turns out that for
![]() , the
standard AMP values of
, the
standard AMP values of ![]() are perfectly stable (up to a
fraction of eV). However, the sum rule, which also tests the
convergence of higher angular momenta, reaches a similar level of
precision only above
are perfectly stable (up to a
fraction of eV). However, the sum rule, which also tests the
convergence of higher angular momenta, reaches a similar level of
precision only above
![]() . The LR values of
. The LR values of ![]() converge only above
converge only above
![]() , which illustrates the fact
that in Eq. (24), higher intermediate angular momenta must be
taken into account. Note, however, that the sum rules calculated
using both methods converge in a similar smooth way.
, which illustrates the fact
that in Eq. (24), higher intermediate angular momenta must be
taken into account. Note, however, that the sum rules calculated
using both methods converge in a similar smooth way.
![\includegraphics[width=0.5\columnwidth]{Reg3aFig2.eps}](img98.png) |
The density-independent Skyrme parametrization SV in its original
formulation [18], that is, without the tensor EDF terms, no
longer corresponds to an interaction. Fig. 2 clearly shows
that even such a seemingly insignificant departure from the true Hamiltonian is immediately detectable through the indicators
tested in our study. In the standard AMP, energy ![]() is again
perfectly stable over the entire range of studied values of
is again
perfectly stable over the entire range of studied values of
![]() . However, such stability can be misleading,
because the LR value, which converges only at
. However, such stability can be misleading,
because the LR value, which converges only at
![]() ,
differs by as much as 2keV.
,
differs by as much as 2keV.
Note that the singularity of energy kernels leaves its fingerprint in
the values of the standard-AMP sum-rule residuals. After an apparent
convergence (at the level of a few keV), which is visible below
![]() , at the level of a few eV, this indicator, in
fact, does not converge to zero. On the other hand, the LR sum-rule
residuals smoothly converge to zero with high precision. An important
conclusion obtained here is that the stability of the ground-state
energy does not necessarily warrant that its value be free from
spurious effects.
, at the level of a few eV, this indicator, in
fact, does not converge to zero. On the other hand, the LR sum-rule
residuals smoothly converge to zero with high precision. An important
conclusion obtained here is that the stability of the ground-state
energy does not necessarily warrant that its value be free from
spurious effects.
![\includegraphics[width=0.5\columnwidth]{Reg3aFig3.eps}](img100.png) |
For the density-dependent SIII functional [18], problems
encountered within the standard AMP are further magnified. In this
example, we performed calculations using the Slater
approximation [25] of the Coulomb exchange energy.
Therefore, here we applied our LR method both to the Skyrme and
Coulomb parts of the functional. The results are depicted in
Fig. 3, showing the energy ![]() (upper panel),
Skyrme-energy sum-rule residuals (middle panel), and Coulomb-energy
sum-rule residuals (lower panel). Similarly as in Fig. 2,
the standard AMP leads to misleadingly stable values of
(upper panel),
Skyrme-energy sum-rule residuals (middle panel), and Coulomb-energy
sum-rule residuals (lower panel). Similarly as in Fig. 2,
the standard AMP leads to misleadingly stable values of ![]() ; however, now
the corresponding sum rules turn out to be completely unstable. For
the Skyrme and Coulomb energies, they stagger around zero at the
level of 50keV and 50eV, respectively. In contrast, the LR method
perfectly stabilizes the sum rules, which smoothly converge to zero,
and leads to stable values of
; however, now
the corresponding sum rules turn out to be completely unstable. For
the Skyrme and Coulomb energies, they stagger around zero at the
level of 50keV and 50eV, respectively. In contrast, the LR method
perfectly stabilizes the sum rules, which smoothly converge to zero,
and leads to stable values of ![]() . However, the LR
. However, the LR ![]() energy is now shifted down by almost 50keV, as compared to the
standard AMP solution.
energy is now shifted down by almost 50keV, as compared to the
standard AMP solution.
![\includegraphics[width=0.5\columnwidth]{Reg3aFig4.eps}](img101.png) |
Finally, let us point out yet another shortcoming of the standard AMP
approach. Fig. 4 shows results of the test of stability of
![]() with respect to the number of mesh points
with respect to the number of mesh points ![]() used in
numerical integrations over the Euler angles. In this example,
calculations were performed for the SIII functional [18] and
exact Coulomb exchange energy. It is clearly visible that the
standard-AMP values of
used in
numerical integrations over the Euler angles. In this example,
calculations were performed for the SIII functional [18] and
exact Coulomb exchange energy. It is clearly visible that the
standard-AMP values of ![]() vary strongly and quite erratically
with
vary strongly and quite erratically
with ![]() . This is owing to the fact that the results do depend on
relative positions of mesh points with respect to singularities of
the energy kernel. In contrast, the LR results are perfectly stable.
. This is owing to the fact that the results do depend on
relative positions of mesh points with respect to singularities of
the energy kernel. In contrast, the LR results are perfectly stable.
Jacek Dobaczewski 2014-12-06