Next: Summary and perspectives Up: Regularization scheme Previous: Linear regularization scheme
In the QR scheme, matrix element
![]() (10) is replaced by the auxiliary
quantity
(10) is replaced by the auxiliary
quantity
![]() defined in Eq. (13) for
defined in Eq. (13) for ![]() .
Again we assume that matrix element
.
Again we assume that matrix element
![]() is regularizable.
Inserting expansions (16) and (20) into (13) gives
is regularizable.
Inserting expansions (16) and (20) into (13) gives
| (32) |
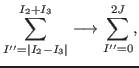 |
(33) |
The QR scheme proposed above is not the simplest one. An alternative
formulation of the scheme, which, in fact, can be generalized to
higher orders, can be obtained as follows. By performing a direct
decomposition of the ![]() th power of the overlap, which is always regular and,
therefore, expandable in terms of the Wigner
th power of the overlap, which is always regular and,
therefore, expandable in terms of the Wigner ![]() -functions, we obtain:
-functions, we obtain:
In this work we used the QR scheme as defined through the equations
(35)-(36). In the applications we used a strategy similar
to the one used for the LR scheme, that is, energies ![]() and
sum-rule residuals were investigated as a functions of
and
sum-rule residuals were investigated as a functions of
![]() , which is the maximum value of angular momentum
admitted in the summations in Eqs. (35) and (36).
, which is the maximum value of angular momentum
admitted in the summations in Eqs. (35) and (36).
![\includegraphics[width=0.5\columnwidth]{Reg3aFig5.eps}](img126.png) |
First, we applied the QR method to the SIII case, already analyzed in
Sec. 2.2 in the context of the LR method, see Fig. 3.
In this case, both regularization schemes are fully equivalent and
give identical results. This result was, in fact, expected. Indeed, let
us observe that regularizability of the theory at certain order ![]() guarantees, see Eq. (17),
that the expression:
guarantees, see Eq. (17),
that the expression:
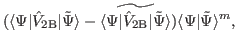 |
(37) |
Next, we applied both regularization schemes to the case of the SLy4
functional [28], which features a fractional-power density
dependence with ![]() . The results are presented in
Fig. 5. In this case, the regularization is insufficient to
stabilize the energy of the lowest
. The results are presented in
Fig. 5. In this case, the regularization is insufficient to
stabilize the energy of the lowest ![]() =0 state. By removing
contributions coming from uncompensated poles in energy kernels, the
QR scheme lowers the energy of the
=0 state. By removing
contributions coming from uncompensated poles in energy kernels, the
QR scheme lowers the energy of the ![]() state as compared to the LR
scheme, but is unable to fully stabilize the solution. The reason is that,
apart from singularities, the fractional power of the density introduces
non-analyticities related to branch cuts, which are not removable by our regularization scheme,
irrespective of its order
state as compared to the LR
scheme, but is unable to fully stabilize the solution. The reason is that,
apart from singularities, the fractional power of the density introduces
non-analyticities related to branch cuts, which are not removable by our regularization scheme,
irrespective of its order ![]() .
.
Jacek Dobaczewski 2014-12-06