


Next: Bibliography
Up: Point symmetries in the
Previous: Conclusions
In this Appendix we explicitly construct irreducible representations
of the D
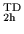 group by using the example of the HO basis
(Sec. 2.4), and we illustrate the Wigner classification of
groups that contain antilinear operators (see Chap. 26 of
Ref. [8]). The results of such an analysis were used in
Sec. 2.
group by using the example of the HO basis
(Sec. 2.4), and we illustrate the Wigner classification of
groups that contain antilinear operators (see Chap. 26 of
Ref. [8]). The results of such an analysis were used in
Sec. 2.
We consider here only the two-dimensional spinor representations,
appropriate for the odd-fermion systems and in particular for
the single-particle states. From Eqs. (47)
one finds representation matrices
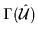 (where operators
(where operators
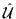
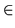 D
D
 of Sec. 2.3 form
the double group D
of Sec. 2.3 form
the double group D
 ), in the two-dimensional invariant subspace spanned
by
), in the two-dimensional invariant subspace spanned
by
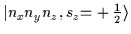 and
and
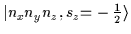 .
We have
.
We have
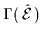 |
= |
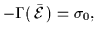 |
(91) |
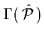 |
= |
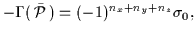 |
(92) |
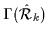 |
= |
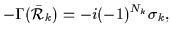 |
(93) |
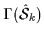 |
= |
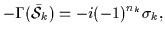 |
(94) |
where 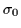 is the identity 2
is the identity 2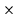 2 matrix,
2 matrix, 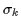 for k=x,y,z are the standard Pauli matrices,
and symbols Nx, Ny, and Nz refer to ny+nz, nx+nz, and
nx+ny, respectively.
for k=x,y,z are the standard Pauli matrices,
and symbols Nx, Ny, and Nz refer to ny+nz, nx+nz, and
nx+ny, respectively.
The characters of the classes are
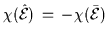 |
= |
2, |
(95) |
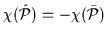 |
= |
2(-1)nx+ny+nz, |
(96) |
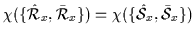 |
= |
0, |
(97) |
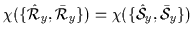 |
= |
0, |
(98) |
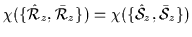 |
= |
0. |
(99) |
One can see, that only the characters of
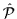 and
and
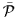 depend on
quantum numbers nx, ny, and nz that define the invariant
subspaces; more precisely, they depend only on the parity of the sum
nx+ny+nz, i.e., on the total parity of basis states. Therefore, the
only two spinor representations of D
depend on
quantum numbers nx, ny, and nz that define the invariant
subspaces; more precisely, they depend only on the parity of the sum
nx+ny+nz, i.e., on the total parity of basis states. Therefore, the
only two spinor representations of D
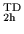 can be labeled by the
eigenvalues of the parity operator
can be labeled by the
eigenvalues of the parity operator
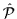 .
Let us also note that all
characters are real.
.
Let us also note that all
characters are real.
If we introduce the time reversal,
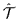 ,
into the ensemble of
the linear operators belonging to D
,
into the ensemble of
the linear operators belonging to D
 we obtain the D
we obtain the D
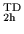 group with 16
new antilinear elements
group with 16
new antilinear elements
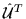
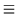
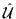
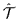 ,
Sec. 2.3.
To study properties of the representations of
the D
,
Sec. 2.3.
To study properties of the representations of
the D
 group, one has to consider representations provided by
matrices
group, one has to consider representations provided by
matrices
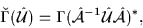 |
(100) |
where
 is one of the antilinear elements of D
is one of the antilinear elements of D
 (see
[8]). It is most convenient to take
(see
[8]). It is most convenient to take
 itself as
itself as
 ;
we then have simply
;
we then have simply
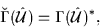 |
(101) |
as
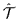 commutes with all
commutes with all
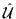
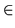 D
D
 .
In such a case, matrices
.
In such a case, matrices
 are just complex conjugates of
are just complex conjugates of
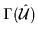 ,
and
therefore the characters of representation
,
and
therefore the characters of representation
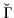 are
exactly the same as those of
are
exactly the same as those of 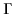 ,
because they are all real,
see Eqs. (95). Therefore these two representations are
equivalent, and a matrix
,
because they are all real,
see Eqs. (95). Therefore these two representations are
equivalent, and a matrix  exists which brings by a similarity
transformation all matrices
exists which brings by a similarity
transformation all matrices
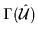 to
to
 =
=
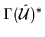 ,
,
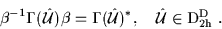 |
(102) |
Now, as shown by Wigner[8], there are only two cases
possible: either
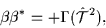 |
(103) |
or
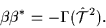 |
(104) |
Matrix  can easily be found from the explicit expressions
for matrices
can easily be found from the explicit expressions
for matrices
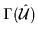 given in Eqs. (91), and it reads
given in Eqs. (91), and it reads
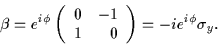 |
(105) |
Choosing the phase factor 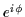
 1 in (105)
is equivalent to a change of phase of the
1 in (105)
is equivalent to a change of phase of the
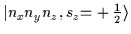 states, and to a change in the phase convention in
Eq. (48).
states, and to a change in the phase convention in
Eq. (48).
It is easy to demonstrate that with this form of the matrix  ,
Eq. (103), and not (104) holds.
In Wigner's classification this case leads to what is called the
corepresentations of the
``first kind'': any representation
,
Eq. (103), and not (104) holds.
In Wigner's classification this case leads to what is called the
corepresentations of the
``first kind'': any representation 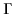 of the group D
of the group D
 can be
completed to a corepresentation of the full D
can be
completed to a corepresentation of the full D
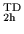 group by defining
group by defining
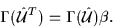 |
(106) |
Note that taking
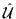 =
=
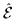 one gets
one gets
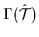 =
= ,
so
,
so  is, of course, just the matrix representing
is, of course, just the matrix representing
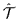 itself.
itself.
After Wigner, the term corepresentation is used here because the
representations of groups containing antilinear operators are
not representations in the usual sense. To see this, let us consider
an orthonormal set of states
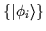 constituting
a basis of a representation
constituting
a basis of a representation 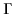 .
Let
.
Let
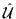 be any linear, and
be any linear, and
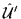 any element of the group. Then, because of antilinearity of
any element of the group. Then, because of antilinearity of
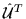 one has
one has
and, consequently,
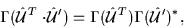 |
(108) |
to be compared with
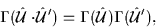 |
(109) |
which holds for the ``usual'' representations.
The presence of complex conjugation on the right-hand
side of Eq. (108) implies that the homomorphism
between the group
multiplication and the multiplication of representation matrices no longer
holds when the group contains antilinear operators.
This is not surprising in view of the fact that matrices,
by construction, always act on vectors (columns of numbers) linearly.
In conclusion, there are only two spinor corepresentations of D
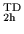 ,
and
they can be labeled, as is also the case for the D
,
and
they can be labeled, as is also the case for the D
 group, by one quantum
number only (parity).
group, by one quantum
number only (parity).



Next: Bibliography
Up: Point symmetries in the
Previous: Conclusions
Jacek Dobaczewski
2000-02-05
![]() group by using the example of the HO basis
(Sec. 2.4), and we illustrate the Wigner classification of
groups that contain antilinear operators (see Chap. 26 of
Ref. [8]). The results of such an analysis were used in
Sec. 2.
group by using the example of the HO basis
(Sec. 2.4), and we illustrate the Wigner classification of
groups that contain antilinear operators (see Chap. 26 of
Ref. [8]). The results of such an analysis were used in
Sec. 2.
![]() (where operators
(where operators
![]()
![]() D
D
![]() of Sec. 2.3 form
the double group D
of Sec. 2.3 form
the double group D
![]() ), in the two-dimensional invariant subspace spanned
by
), in the two-dimensional invariant subspace spanned
by
![]() and
and
![]() .
We have
.
We have
![]() ,
into the ensemble of
the linear operators belonging to D
,
into the ensemble of
the linear operators belonging to D
![]() we obtain the D
we obtain the D
![]() group with 16
new antilinear elements
group with 16
new antilinear elements
![]()
![]()
![]()
![]() ,
Sec. 2.3.
To study properties of the representations of
the D
,
Sec. 2.3.
To study properties of the representations of
the D
![]() group, one has to consider representations provided by
matrices
group, one has to consider representations provided by
matrices
![]() ,
Eq. (103), and not (104) holds.
In Wigner's classification this case leads to what is called the
corepresentations of the
``first kind'': any representation
,
Eq. (103), and not (104) holds.
In Wigner's classification this case leads to what is called the
corepresentations of the
``first kind'': any representation ![]() of the group D
of the group D
![]() can be
completed to a corepresentation of the full D
can be
completed to a corepresentation of the full D
![]() group by defining
group by defining
![]() constituting
a basis of a representation
constituting
a basis of a representation ![]() .
Let
.
Let
![]() be any linear, and
be any linear, and
![]() any element of the group. Then, because of antilinearity of
any element of the group. Then, because of antilinearity of
![]() one has
one has
![]() ,
and
they can be labeled, as is also the case for the D
,
and
they can be labeled, as is also the case for the D
![]() group, by one quantum
number only (parity).
group, by one quantum
number only (parity).