


Next: Axially deformed harmonic oscillator
Up: Transformed Harmonic Oscillator Basis
Previous: Transformed harmonic oscillator wave
In principle, we could use the flexibility of having three different LST
functions fk(r) and three different oscillator lengths Lk of the original
deformed HO basis to tailor the LST transformation to the shape of the deformed
nucleus under investigation. However, for large HO bases (in the present study we
include HO states up to 20 major shells), the dependence of the total energy on
the basis deformation is very weak, so that minimization of the total energy with
respect to the three oscillator lengths Lk is ill-conditioned (see discussion
and examples given in Ref. [15]). Therefore, in this study, we use a
spherical HO basis depending on a single common oscillator length L0,
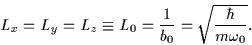 |
(13) |
With such a choice, it is natural to set the three LST functions fk(r) equal
to one another,
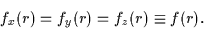 |
(14) |
This allows us to use exactly the same LST function
f(r) as in the previous studies [10,11]. Under conditions
(14), the Jacobian (3) assumes the simpler form
| D |
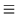 |
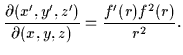 |
(15) |
The parametrization of the LST function f(r) used in
Refs. [10,11] was of the form
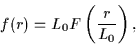 |
(16) |
with the dimensionless
universal function F of the dimensionless variable 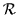 defined as
defined as
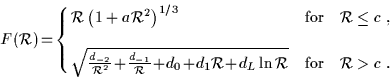 |
(17) |
Two different formulae can be
obtained for the function
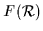 ,
one for
,
one for
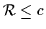 and one for
and one for
 .
Imposing the condition that the function should be continuous at
the matching radius c and that it should have continuous first, second,
third, and fourth derivatives leads to the following requirements for the
constants d-2, d-1, d0, d1, and dL:
.
Imposing the condition that the function should be continuous at
the matching radius c and that it should have continuous first, second,
third, and fourth derivatives leads to the following requirements for the
constants d-2, d-1, d0, d1, and dL:
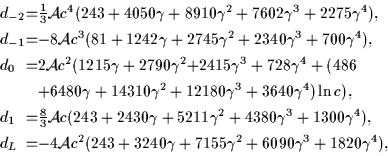 |
(18) |
where 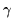 =ac2 and
=ac2 and
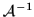 =
=
 .
In this way, the LST function f(r) is
guaranteed to be very smooth, while still depending on only three parameters,
L0, a and c.
.
In this way, the LST function f(r) is
guaranteed to be very smooth, while still depending on only three parameters,
L0, a and c.
From (17), we see that asymptotically the function
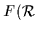
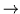
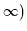
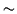
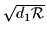 .
Thus, the LST function
obeys conditions (11) provided that the parameters satisfy
.
Thus, the LST function
obeys conditions (11) provided that the parameters satisfy
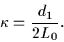 |
(19) |
Two
different approaches can be used in calculations. One possibility is to minimize
the total energy with respect to L0, a, and c, obtaining as output the
energetically optimal value of the decay constant 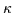 .
Alternatively, for a
given choice of
.
Alternatively, for a
given choice of 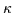 ,
we could eliminate one of the three parameters and
minimize the total energy with respect to the other two. The actual procedure
used in our calculations is described in Sec. 4.1.
,
we could eliminate one of the three parameters and
minimize the total energy with respect to the other two. The actual procedure
used in our calculations is described in Sec. 4.1.



Next: Axially deformed harmonic oscillator
Up: Transformed Harmonic Oscillator Basis
Previous: Transformed harmonic oscillator wave
Jacek Dobaczewski
1999-09-13
![]()
![]()
![]()
![]()
![]() .
Thus, the LST function
obeys conditions (11) provided that the parameters satisfy
.
Thus, the LST function
obeys conditions (11) provided that the parameters satisfy