


Next: Conserved symmetries
Up: Local Density Approximation for
Previous: The P-H and P-P
The HFB equations
Minimization of the energy functional of Eq. (72) with
respect to the p-h and p-p density matrices, which fulfill
Eqs. (13) under auxiliary conditions
leads to the HFB equation of the form:
![\begin{displaymath}
\left[\hat{\breve{\mathcal H}},\hat{\breve{\mathcal R}}\right] =0.
\end{displaymath}](img604.png) |
(198) |
The generalized density matrix
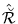 is given by Eq. (16) and the generalized mean-field Hamiltonian is defined
as
is given by Eq. (16) and the generalized mean-field Hamiltonian is defined
as
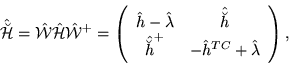 |
(199) |
with the Lagrange multiplier given by
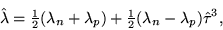 |
(200) |
where 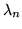 and
and 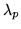 are the neutron and proton Fermi
levels, respectively.
are the neutron and proton Fermi
levels, respectively.
The usual method of solving the HFB equation (199) is to solve
in a self-consistent way the eigenvalue problem,
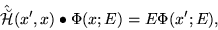 |
(201) |
for the generalized mean-field Hamiltonian, and then to construct the
generalized density matrix,
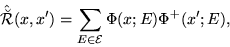 |
(202) |
as a projection operator onto the set of the quasihole (occupied)
states  belonging to a subset of energy spectrum,
belonging to a subset of energy spectrum, 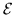 .
For a local mean-field Hamiltonian, Eq. (202) is a system of
eight second-order differential equations, in
general with complex coefficients. Usual four dimensions corresponding
to upper and lower HFB components and to two spin projections are
here multiplied by another factor of two due to the isospin projections.
Altogether, Eq. (202) corresponds to a system of
sixteen equations within the domain of real numbers. When specific
symmetry conditions are imposed on solutions, this number can be reduced
in a standard way, see Ref. [184] for the analysis
pertaining to spherical symmetry.
.
For a local mean-field Hamiltonian, Eq. (202) is a system of
eight second-order differential equations, in
general with complex coefficients. Usual four dimensions corresponding
to upper and lower HFB components and to two spin projections are
here multiplied by another factor of two due to the isospin projections.
Altogether, Eq. (202) corresponds to a system of
sixteen equations within the domain of real numbers. When specific
symmetry conditions are imposed on solutions, this number can be reduced
in a standard way, see Ref. [184] for the analysis
pertaining to spherical symmetry.
The energy spectrum of generalized mean-field Hamiltonian has been
discussed in Ref. [5]. The only difference with the
present case is that here the eigenvalue problems for neutrons and
protons in Eq. (202) cannot be separated. It is well known,
that the eigenvalues of
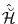 appear in pairs of
opposite signs. For each quasihole state of energy
appear in pairs of
opposite signs. For each quasihole state of energy 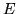
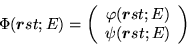 |
(203) |
there exists a quasiparticle state
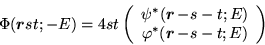 |
(204) |
belonging to energy 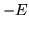 . In the case of absence of external fields,
bound states (when
. In the case of absence of external fields,
bound states (when 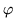 and
and 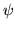 are both localized) exist only
when both Fermi levels,
are both localized) exist only
when both Fermi levels, 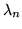 and
and 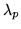 , are negative.
Discrete quasihole energy levels lie within the range
, are negative.
Discrete quasihole energy levels lie within the range
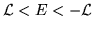 , where
, where
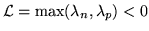 . The ground-state solution corresponds
to occupying states having negative energies; then the set
. The ground-state solution corresponds
to occupying states having negative energies; then the set  consists of a number of discrete levels lying inside segment
consists of a number of discrete levels lying inside segment
 and the continuous spectrum with
and the continuous spectrum with
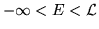 .
.
Traditionally, one solves Eq. (202) for the quasiparticle states
of positive energies rather than for the negative ones. Then, the
discrete spectrum is within the segment
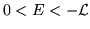 and energies
and energies
 belong to the continuum. Having found the
wavefunctions
belong to the continuum. Having found the
wavefunctions
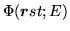 for
for 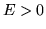 one uses
Eq. (205) to construct the density matrix, i.e.,
one uses
Eq. (205) to construct the density matrix, i.e.,
 |
(205) |
The p-h and p-p density matrices are then expressed as



Next: Conserved symmetries
Up: Local Density Approximation for
Previous: The P-H and P-P
Jacek Dobaczewski
2004-01-03
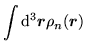
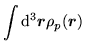
![]() appear in pairs of
opposite signs. For each quasihole state of energy
appear in pairs of
opposite signs. For each quasihole state of energy ![]()
![]() and energies
and energies
![]() belong to the continuum. Having found the
wavefunctions
belong to the continuum. Having found the
wavefunctions
![]() for
for ![]() one uses
Eq. (205) to construct the density matrix, i.e.,
one uses
Eq. (205) to construct the density matrix, i.e.,