Simple regularization scheme for multi-reference density functional theories

W. Satula, J. Dobaczewski

Date: December 6, 2014

Abstract:

Background

Extensions of single-reference (SR) energy-density-functionals (EDFs) to multi-reference (MR) applications involve using the generalized Wick theorem (GWT), which leads to singular energy kernels that cannot be properly integrated to restore symmetries, unless the EDFs are generated by true interactions.

Purpose

We propose a new method to regularize the MR EDFs, which is based on using auxiliary quantities obtained by multiplying the kernels with appropriate powers of overlaps.

Methods

Regularized matrix elements of two-body interactions are obtained by integrating the auxiliary quantities and then solving simple linear equations.

Results

We implement the new regularization method within the self-consistent Skyrme-Hartree-Fock approach and we perform a proof-of-principle angular-momentum projection (AMP) of states in odd-odd nucleus 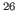$^{26}$Al. We show that for EDFs generated by true interactions, our regularization method gives results identical to those obtained within the standard AMP procedure. We also show that for EDFs that do not correspond to true interactions, it gives stable and converging results that are different than unstable and non-converging standard AMP values.

Conclusions

The new regularization method proposed in this work may provide us with a relatively inexpensive and efficient tool to generalize SR EDFs to MR applications, thus allowing for symmetry restoration and configuration mixing performed for typical nuclear EDFs, which most often do not correspond to true interactions.