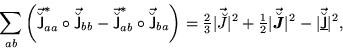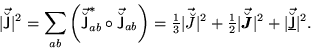Next: The P-H and P-P
Up: Skyrme interaction energy functional
Previous: The p-h channel
The p-p channel
In the p-p energy density, each operator of the relative
momentum,
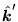 and
and
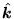 , acts on variables
of the same density matrix, and thus no recoupling is necessary.
Terms of the interaction that are linear in momenta then lead to
current densities (47) and (51), while terms
that are quadratic in momenta lead to derivatives of local densities
and to kinetic densities (41), (45), and
(49), because
, acts on variables
of the same density matrix, and thus no recoupling is necessary.
Terms of the interaction that are linear in momenta then lead to
current densities (47) and (51), while terms
that are quadratic in momenta lead to derivatives of local densities
and to kinetic densities (41), (45), and
(49), because
However, in the p-p energy density, indices of Pauli matrices
couple together the two density matrices, and hence do require recoupling
to the p-p channel. These recoupling formulae can be obtained by means of
the standard algebra of angular momentum. A sum of the three Clebsch-Gordan
coefficients appropriate to the present case
reads [179]
| |
|
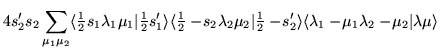 |
|
| |
|
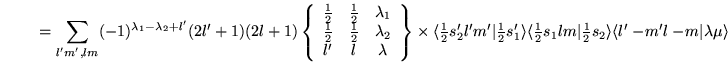 |
(144) |
Taking relevant combinations of
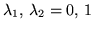 and
and
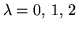 , one obtains:
, one obtains:
and the formulae similar to Eqs. (145) and (146)
are obtained for
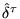 and
and
 , respectively.
, respectively.
The two zero-order (density-dependent) p-p coupling constants
of the energy density (80) are related to the Skyrme
parameters in the following way:
and the second-order p-p coupling constants are given in Table
2. Similar to the p-h case, the gauge-invariance conditions
(112) are met.
Table 2:
Second-order coupling constants of the p-p energy density
(80) as functions of parameters of the Skyrme
interaction (117), expressed by the formula:
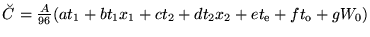 .
.
| |
 |
|
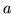 |
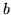 |
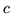 |
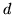 |
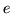 |
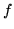 |
 |
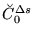 |
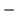 3 3 |
|
1 |
1 |
0 |
0 |
2 |
0 |
0 |
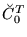 |
12 |
|
1 |
1 |
0 |
0 |
2 |
0 |
0 |
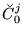 |
12 |
|
0 |
0 |
1 |
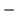 1 1 |
0 |
0 |
0 |
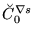 |
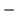 18 18 |
|
0 |
0 |
0 |
0 |
1 |
0 |
0 |
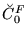 |
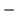 72 72 |
|
0 |
0 |
0 |
0 |
1 |
0 |
0 |
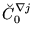 |
0 |
|
0 |
0 |
0 |
0 |
0 |
0 |
0 |
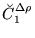 |
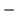 3 3 |
|
1 |
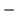 1 1 |
0 |
0 |
0 |
0 |
0 |
 |
12 |
|
1 |
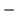 1 1 |
0 |
0 |
0 |
0 |
0 |
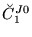 |
4 |
|
0 |
0 |
1 |
1 |
0 |
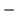 10 10 |
4 |
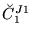 |
6 |
|
0 |
0 |
1 |
1 |
0 |
5 |
2 |
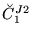 |
12 |
|
0 |
0 |
1 |
1 |
0 |
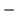 1 1 |
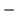 2 2 |
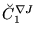 |
0 |
|
0 |
0 |
0 |
0 |
0 |
0 |
0 |
Equivalently, the density-dependent zero-range pairing force
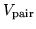 can be used in the p-p channel
[180,181,182,183],
can be used in the p-p channel
[180,181,182,183],
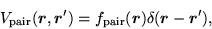 |
(151) |
for
![\begin{displaymath}
f_{\mbox{\rm\scriptsize {pair}}}(\mbox{{\boldmath {$r$}}}) =...
...$r$}}})}{\rho_c}\right]^\alpha
(1+x_3\hat{P}^\sigma)\right\} ,
\end{displaymath}](img473.png) |
(152) |
where
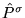 is the spin-exchange operator (120).
In such a case, coupling constants (149) read
is the spin-exchange operator (120).
In such a case, coupling constants (149) read
Note that when only the isovector pairing is used, as in most LDA
applications to date, the exchange parameters 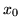 and
and 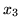 are
redundant in the definition of the isovector coupling constant
are
redundant in the definition of the isovector coupling constant
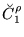 , and hence are usually set to 0. However, if one
wants to independently model the isoscalar and isovector pairing
intensity, one has to use non-zero values of
, and hence are usually set to 0. However, if one
wants to independently model the isoscalar and isovector pairing
intensity, one has to use non-zero values of 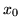 and
and 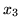 .
.
For the Gogny interaction [167], the zero-range
density-dependent term 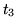 with
with 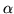 =1/3 was used in order to
enforce proper saturation properties. The corresponding exchange
parameter
=1/3 was used in order to
enforce proper saturation properties. The corresponding exchange
parameter 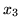 =1 was used to prevent this zero-range force from
contributing to the isovector pairing channel. However, such a
choice, when applied literally to the proton-neutron mixing case,
might lead to a very strong repulsive isoscalar pairing interaction.
=1 was used to prevent this zero-range force from
contributing to the isovector pairing channel. However, such a
choice, when applied literally to the proton-neutron mixing case,
might lead to a very strong repulsive isoscalar pairing interaction.
The term of  coming from the spin-orbit interaction
contains the combination of components of the p-p spin-current
density
coming from the spin-orbit interaction
contains the combination of components of the p-p spin-current
density
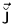 ,
,
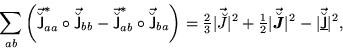 |
(155) |
that is different from that coming from the tensor
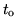 term,
term,
| |
|
 |
|
| |
|
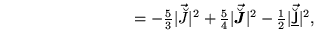 |
(156) |
and from that coming from the central 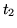 term,
term,
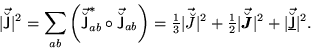 |
(157) |
Therefore, by setting appropriate values of the 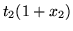 ,
, 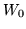 ,
and
,
and
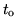 parameters, one can obtain arbitrary values of the
spin-current coupling constants
parameters, one can obtain arbitrary values of the
spin-current coupling constants
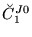 ,
,
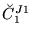 , and
, and
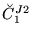 . Similarly, parameter
. Similarly, parameter
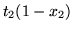 allows for fixing an arbitrary value of the current coupling
constant
allows for fixing an arbitrary value of the current coupling
constant
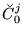 . On the other hand, parameter
. On the other hand, parameter
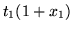 defines two isoscalar coupling constants,
defines two isoscalar coupling constants,
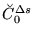 and
and
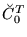 , parameter
, parameter
 defines another two isoscalar coupling constants,
defines another two isoscalar coupling constants,
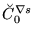 and
and
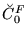 , and parameter
, and parameter
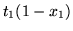 defines two isovector coupling constants,
defines two isovector coupling constants,
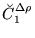 and
and
 ; hence, these
pairs of coupling constants are not independent from one another.
These three pairs of dependencies reflect, in fact, the three
gauge invariance conditions (104).
In this way, seven Skyrme force parameters determine ten coupling constants
in the p-p channel.
Finally, the Skyrme interaction does not give any non-zero values for
the spin-orbit coupling constants
; hence, these
pairs of coupling constants are not independent from one another.
These three pairs of dependencies reflect, in fact, the three
gauge invariance conditions (104).
In this way, seven Skyrme force parameters determine ten coupling constants
in the p-p channel.
Finally, the Skyrme interaction does not give any non-zero values for
the spin-orbit coupling constants
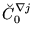 and
and
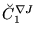 . Therefore, up to the gauge invariance
conditions, the Skyrme interaction fully determines the energy
density in the p-p channel.
. Therefore, up to the gauge invariance
conditions, the Skyrme interaction fully determines the energy
density in the p-p channel.



Next: The P-H and P-P
Up: Skyrme interaction energy functional
Previous: The p-h channel
Jacek Dobaczewski
2004-01-03
![]() and
and
![]() , acts on variables
of the same density matrix, and thus no recoupling is necessary.
Terms of the interaction that are linear in momenta then lead to
current densities (47) and (51), while terms
that are quadratic in momenta lead to derivatives of local densities
and to kinetic densities (41), (45), and
(49), because
, acts on variables
of the same density matrix, and thus no recoupling is necessary.
Terms of the interaction that are linear in momenta then lead to
current densities (47) and (51), while terms
that are quadratic in momenta lead to derivatives of local densities
and to kinetic densities (41), (45), and
(49), because
![]() can be used in the p-p channel
[180,181,182,183],
can be used in the p-p channel
[180,181,182,183],
![]() with
with ![]() =1/3 was used in order to
enforce proper saturation properties. The corresponding exchange
parameter
=1/3 was used in order to
enforce proper saturation properties. The corresponding exchange
parameter ![]() =1 was used to prevent this zero-range force from
contributing to the isovector pairing channel. However, such a
choice, when applied literally to the proton-neutron mixing case,
might lead to a very strong repulsive isoscalar pairing interaction.
=1 was used to prevent this zero-range force from
contributing to the isovector pairing channel. However, such a
choice, when applied literally to the proton-neutron mixing case,
might lead to a very strong repulsive isoscalar pairing interaction.
![]() coming from the spin-orbit interaction
contains the combination of components of the p-p spin-current
density
coming from the spin-orbit interaction
contains the combination of components of the p-p spin-current
density
![]() ,
,